- 7.57 MB
- 2022-04-29 14:01:04 发布
- 1、本文档共5页,可阅读全部内容。
- 2、本文档内容版权归属内容提供方,所产生的收益全部归内容提供方所有。如果您对本文有版权争议,可选择认领,认领后既往收益都归您。
- 3、本文档由用户上传,本站不保证质量和数量令人满意,可能有诸多瑕疵,付费之前,请仔细先通过免费阅读内容等途径辨别内容交易风险。如存在严重挂羊头卖狗肉之情形,可联系本站下载客服投诉处理。
- 文档侵权举报电话:19940600175。
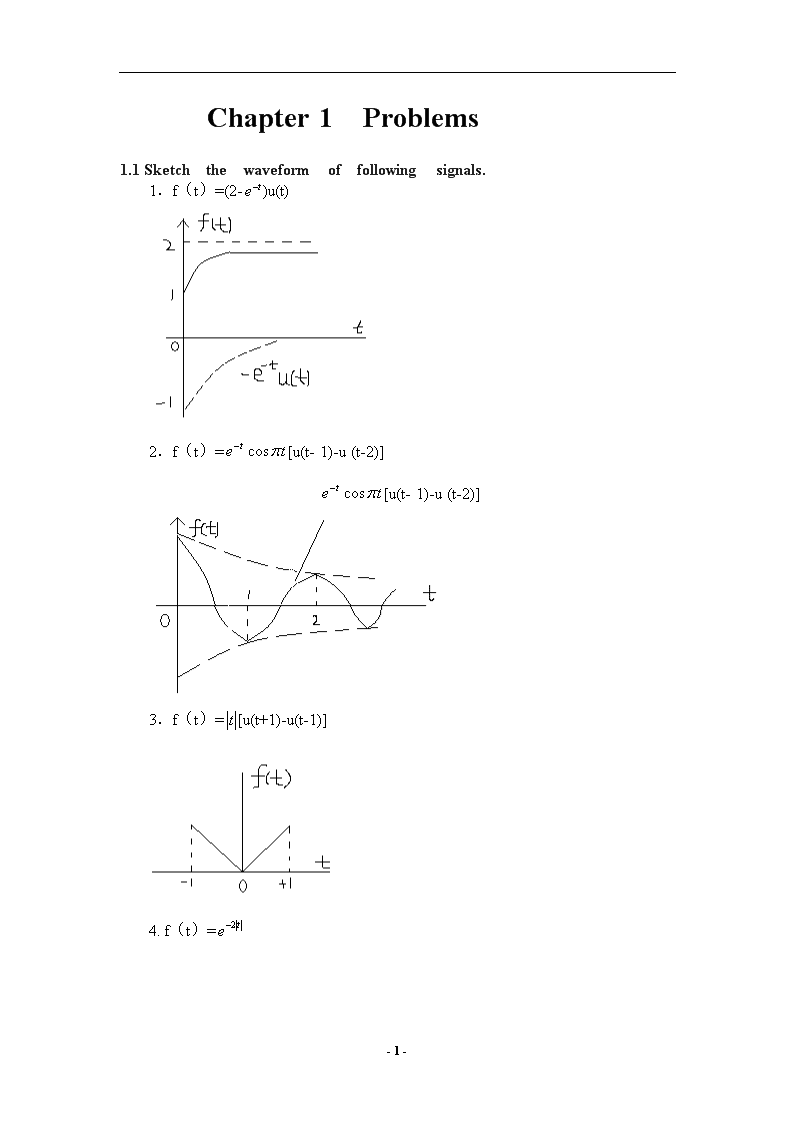
'Chapter1Problems1.1Sketchthewaveformoffollowingsignals.1.f(t)=(2-)u(t)2.f(t)=[u(t-1)-u(t-2)][u(t-1)-u(t-2)]3.f(t)=[u(t+1)-u(t-1)]4.f(t)=-78-
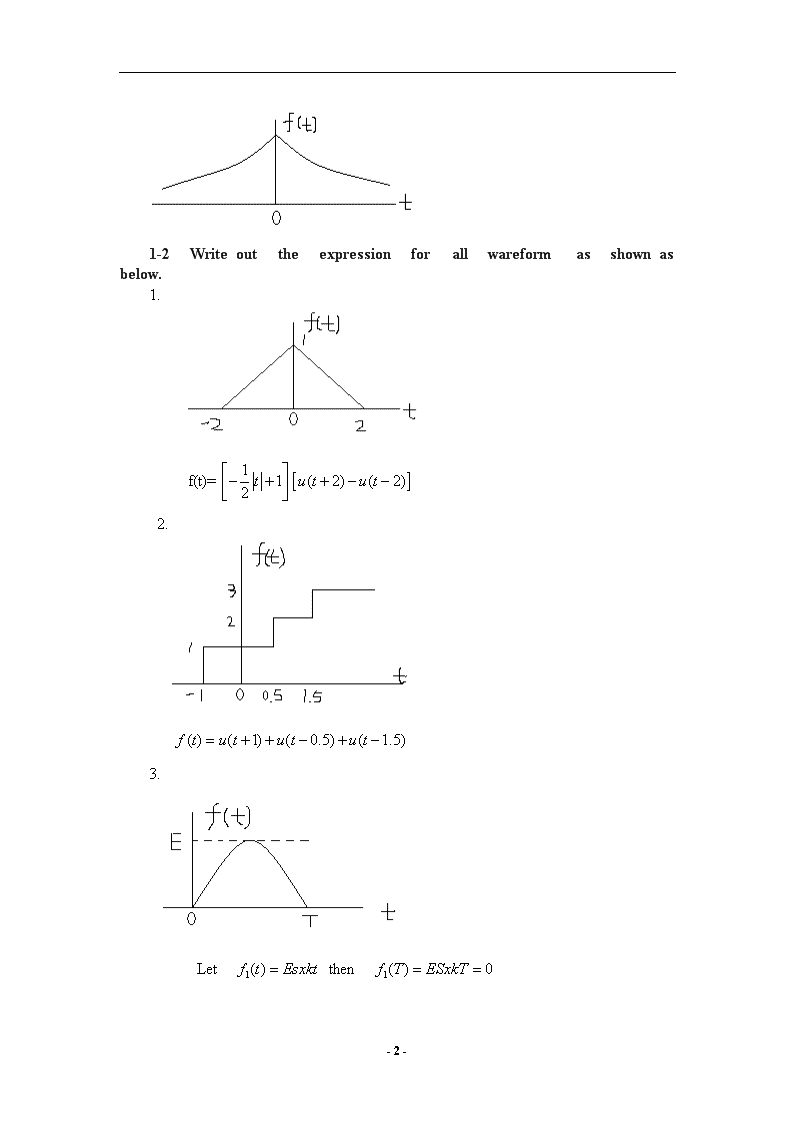
1-2Writeouttheexpressionforallwareformasshownasbelow.1.f(t)=2.3.Letthen-78-
SothatkT==1-3Determingwhetherthe1,cost,…,cosntareorthogonalfunctiononinterval(0,2).Proof:=1.4Determingwhether1,x,,areorthogonalfunctionontheinterval[0.1].Proof:Sothatontheinterval[0.1]theyarenotorthogonal.1-5Findthevalueoftheintegral.1.2.34.5.-78-
6.1-6Thewareformoff(t)isdepictedasbelow,pleasesketchthewaveformoff(t-3),f(-2t-1).Solution:f(t-3)f(-2t-1)1-7f(t)asshownasFig.Tryingtosketchthewaveformoffollowingsignals.-78-
1.f(3t)2.f()u(3-t)3.4.1.8Plotthewareformofgivendiscretesignals.-78-
1.2.3.4.5.-78-
1-9Calculatetheconvolutionofand1.=u(t),=u(t)*====tu(t)2.=u(t),=*=====3.==4.,-78-
====1-10Determingtheperiodicforthefollowingsignals.(1)Let===X(n)SotheperrodisN=40(2)Let==SotheperiodisN=801-11Findthewaveformofthef1【(t)*f2(t)(a)Solution:f1(t)=u(t)–u(t-1),f2(t)=t[u(t)–u(t-2)]f1(t)*f2(t)=f2()f(t-)d=[u(z)-u(-2)][u(t-2)-u(t--1)]d={du(t)-du(t-1)-du(t-2)+-78-
du(t-3)}=[u(t)-u(t-1)-(-2)u(t-2)]+[-2]u(t-3)OrcalculatebymeansofgraghWesee(1)02x(n)isrightsequencex(n)=[]u(n)(2)|z|<0.5x(n)isleftsequencex(n)=[]u(-n-1)(3)0.5<|z|<2x(n)isbilateralsequencex(n)=u(n)+u(-n-1)-78-
Chapter5Problems5.1categorizeeachofthefollowingsignalasanenergysignalsorapowersignals,andfindtheenergyortime-averagedpowerofthesignals.(1)(2)f(t)=5cosπt+sin5πt(3)Solution:(1)f(t)isenergysignalandit’senergyisE=f(t)d(t)=tdt+(2-t)dt=(1/3)t|+(1/3)(2-t)|=1/3+(1/3)(1-0)=2/3(2)f(t)ispowersignalandit’spowerisP=(1/T)f(t)dt=(1/T)(25cosπt+sin5πt+10cosπtsin5πt)dt=(1/T)(25/2+(1/2)cos2πt+1/2+(1/2)cos10πt)dt=(1/T)13dt=13(3)f(t)isenergysignalandit’senergyisE=f(t)dt=25cosπtdt=25(1/2+(1/2)cos2πt)dt=25/2(where=2π/TT=1)5.2Findthepowerofthesignalandplotthespectrumforenergyone,thesignalaregivenasbellow.1.Acos2000πt+Bsin200πt2.(A+sin200πt)cos2000πt-78-
3.Asin(200πt)cos(2000πt)4.Asin(200πt)cos(2000πt)Solution:Let=2000πtΩ=200πt,thentheallofsignalsaremodulatedsignalsexcept1.1.Acost+BsinΩt=(A/2)e+(A/2)e+(B/2j)e+(B/2j)eS(w)=2π=2π[(A/4)+(A/4)+(B/4)+(B/4)]P=(1/2π)=A/2+B/22.(A+sin200πt)cos2000πt=(A+sint)cost=cost+sintcost=(A/2)e+(A/2)e+(1/2)sin(+)+(1/2)sin(-)=(A/2)e+(A/2)e+(1/4)e+(1/4)e+(1/4)e+(1/4)eS()=2π[(A/4)+(A/4)+(1/16)+(1/16)+(1/16)+(1/16)P=(1/2π)=(A/4)+(A/4)+4×(1/16)=(A/2)+1/4=(2A+1)/43.Asin(200πt)cos(2000πt)=Asin(Ωt)cost=[A/2-(A/2)cos2Ωt]cost=(A/2)cost-(A/2)costcos2Ωt=(A/4)e+(A/4)e-(A/4)[cos(+2Ω)t+cos(-2Ω)t]=(A/4)e+(A/4)e-(A/8)e-(A/8)e-78-
-(A/8)e-(A/8)eS(w)=(A/16)+(A/16)+(A/64)+(A/64)+(A/64)+(A/64)P=(1/2π)=(A/8)+(A/32)+(A/32)=(A/8)+(A/16)=3A/164.Asin(200πt)cos(2000πt)=Asin(Ωt)cost=AsinΩtsinΩt+cost=(A/2-A/2cos2Ωt)sinΩtcost=(A/2)sinΩtcost-(A/2)cos2ΩtsinΩtcost=(A/2)[(e-e)/2j]×[(e+e)/2]-(A/2)[(e+e)/2×(e-e)/2j](e+e)/2=(A/8j)[e+e-e-e]+Aj/8[e-e+e-e][(e+e)/2]=(A/8j)[e+e-e-e]+Aj/16[e-e+e-e+e-e+e-e]S(w)=2π[(A/64)(+++)]+2π(A/16){+++++++}P=(1/2π)=(A/64)×4+(A/16)×8=(A/16)+(A/32)=3A/325.3Determinetheenergyspectrumforgivensignals.-78-
F(t)=E×eu(t)Solution:E==E×(1/2a)e|=E/2a5.4Findtheautocorrelationfunctionfortriangularpole.Solution:R(z)=f(t)=-At/T+A=A[1-t/T]ForT+≤0R(z)=0or<-T>TR(z)=0AndforT+>0andT+0and
您可能关注的文档
- 《传统文化与法制中国》测试题满分答案.docx
- 《传统文化与法制中国》真题(附答案).doc
- 《低碳经济》试题答案合集 扬州市专业技术人员.docx
- 《低碳经济与幸福指数》练习题及参考答案.doc
- 《低碳经济循环经济与加快经济发展方式转变》考试题库及参考答案.doc
- 《供配电技术》唐志平第三版习题答案(全).doc
- 《保险会计》习题及答案.doc
- 《保险学教程》各章练习题答案.doc
- 《保险学概论》练习题及答案大全.doc
- 》第二版课后答案_(郑君里)_高等教育出版社.pdf
- 《信号与线性系统》试题与答案6.doc
- 《信息安全原理与技术》(第2版)习题答案.doc
- 《信息安全技术》习题及答案(最新最全).doc
- 《信息理论与编码》第二版( 王虹)课后习题答案.pdf
- 《信息论、编码与密码学》课后习题答案.doc
- 《信息论基础》第一版习题解答.pdf
- 《健康教育》知识点及试题精粹答案.doc
- 《光纤通信》试卷题及答案.docx